“Cooling rate”的版本间差异
(创建页面,内容为“Cooling rates as a function of temperature for a primordial gas composed of atomic hydrogen and helium, as well as molecular hydrogen, in the absence of any external...”) |
无编辑摘要 |
||
| (未显示同一用户的1个中间版本) | |||
| 第1行: | 第1行: | ||
[[File: Cooling_curve.jpg | cooling rate]] |
|||
credit: http://ned.ipac.caltech.edu/level5/Sept06/Loeb/Loeb5.html |
|||
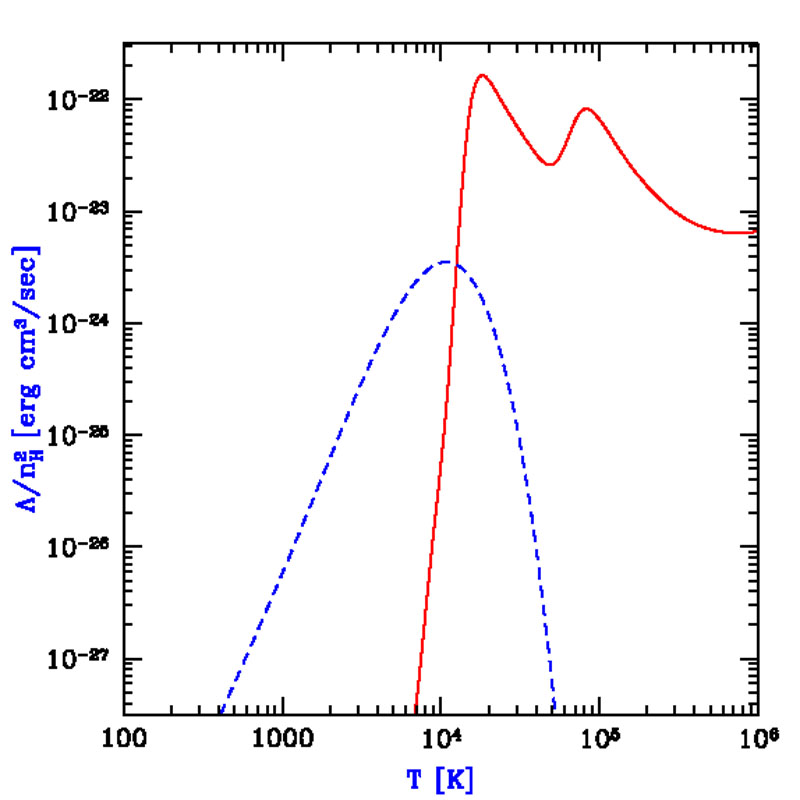
Cooling rates as a function of temperature for a primordial gas composed of atomic hydrogen and helium, as well as molecular hydrogen, in the absence of any external radiation. We assume a hydrogen number density nH = 0.045 cm-3, corresponding to the mean density of virialized halos at z = 10. The plotted quantity Lambda / nH2 is roughly independent of density (unless nH > 10 cm-3), where Lambda is the volume cooling rate (in erg/sec/cm3). The solid line shows the cooling curve for an atomic gas, with the characteristic peaks due to collisional excitation of H1 and He2. The dashed line shows the additional contribution of molecular cooling, assuming a molecular abundance equal to 1% of nH. |
Cooling rates as a function of temperature for a primordial gas composed of atomic hydrogen and helium, as well as molecular hydrogen, in the absence of any external radiation. We assume a hydrogen number density nH = 0.045 cm-3, corresponding to the mean density of virialized halos at z = 10. The plotted quantity Lambda / nH2 is roughly independent of density (unless nH > 10 cm-3), where Lambda is the volume cooling rate (in erg/sec/cm3). The solid line shows the cooling curve for an atomic gas, with the characteristic peaks due to collisional excitation of H1 and He2. The dashed line shows the additional contribution of molecular cooling, assuming a molecular abundance equal to 1% of nH. |
||
2016年1月20日 (三) 01:34的最新版本
credit: http://ned.ipac.caltech.edu/level5/Sept06/Loeb/Loeb5.html
Cooling rates as a function of temperature for a primordial gas composed of atomic hydrogen and helium, as well as molecular hydrogen, in the absence of any external radiation. We assume a hydrogen number density nH = 0.045 cm-3, corresponding to the mean density of virialized halos at z = 10. The plotted quantity Lambda / nH2 is roughly independent of density (unless nH > 10 cm-3), where Lambda is the volume cooling rate (in erg/sec/cm3). The solid line shows the cooling curve for an atomic gas, with the characteristic peaks due to collisional excitation of H1 and He2. The dashed line shows the additional contribution of molecular cooling, assuming a molecular abundance equal to 1% of nH.